A.Mary Selvam and A.S.R.Murty
Indian Institute of Tropical Meteorology,
Pune 411 008, India
Proc. Int'l. Conf. on Energy Transformations and Interactions with Small and Meso-scale Atmospheric Processes, 2 - 6 March, 1987, Switzerland.
(Retired) email: selvam@ip.eth.net
web site: http://www.geocities.com/amselvam
Abstract
A scale invariant,
selfsimilar atmospheric eddy continuum exists in the planetary atmospheric
boundary layer spanning several orders of magnitude in scales and gives
rise to the observed fractal geometry for the global cloud cover pattern.
The global weather systems are manifestations of the unified atmospheric
eddy continuum with inherent mutual global-local energy exchange and therefore
local urban energy/pollution sources have long-range global effects leading
to climate change and environmental degradation. It is shown that the observed
scale invariant atmospheric eddy continuum originates from the turbulence
scale by the universal period doubling route to chaos eddy growth phenomenon
in the planetary atmospheric boundary layer. The cloud dynamical, microphysical
and electrical parameters are shown to be simple unique functions of turbulence
scale energy generation.
1. Introduction
The weather systems
in the downwind of large industrial complexes are known to be affected
by the large quantities of waste heat, moisture, particulate matter and
gaseous pollutants released into the atmosphere from the stacks of industries(
Mathews et al., 1971 ). Investigations relating to the interactions
of several atmospheric processes are important for climate related studies.
Recent studies have indicated selfsimilarity in atmospheric processes and
its application for physical processes in the prediction of climate( Mary
Selvam, 1986 ). Governing equations for atmospheric dynamics have been
derived and it has been shown that the meso-scale cloud clusters( MCC )
evolve from a semi-permanent hierarchical atmospheric eddy continuum whose
energy structure obeys laws analogous to quantum mechanical laws for the
subatomic dynamics. There is selfsimilarity in the dynamical properties
of turbulent, convective, meso-, synoptic and planetary scales and a red
energy cascade takes place from the turbulence to planetary scales ( Mary
Selvam et al.,1984a ). Observations of thermodynamic properties
in the troposphere and stratosphere and measurements of plasma irregularities
in the ionosphere and the magnetosphere indicate the existence of a continuous
spectrum of eddies following a power law n-n
where n
is the frequency and n is the exponent ( Lovejoy and Schertzer,
1986 ). Hence, for the understanding of the physical processes relating
to the urban effects on climate related processes, investigations relating
to the dynamics of the atmospheric boundary layer, cloud physical aspects
and the coupling of the troposphere - stratosphere - ionosphere are important.
These aspects are briefly discussed in this paper.
2.0 Cloud Model
In general, convective
elements in the atmospheric planetary boundary layer ( PBL ) are
frequently organised in bands ( Eymard, 1985 ) indicating the existence
of helical vortex rolls ( large eddy ) circulations in the PBL .
It is not clear how such well-organised long-lived circulations are maintained
in the dissipative turbulent environment of the PBL ( Tennekes,
1973 ). In the following model it is shown that, on the contrary, turbulence
of surface frictional origin maintains a semi-permanent hierarchical continuum
eddy structure in the PBL .
The mean flow generates
turbulent eddies with a net upward motion at the planetary surface by friction
caused by natural and man-made topography and vegetation cover. The incessant
turbulence scale upward momentum is progressively amplified by decrease
of atmospheric density with height coupled with buoyant energy production
from microscale - fractional - condensation ( MFC ) by deliquescence
on hygroscopic nuclei even in an unsaturated environment ( Pruppacher and
Klett, 1978 ). A large scale upward motion is imparted to the mean
flow by the turbulence scale upward momentum flux and gives rise to the
generation of large eddies or vortex roll circulations in the PBL
. A conceptual model of the large eddies in the PBL is given at
Figure 1.
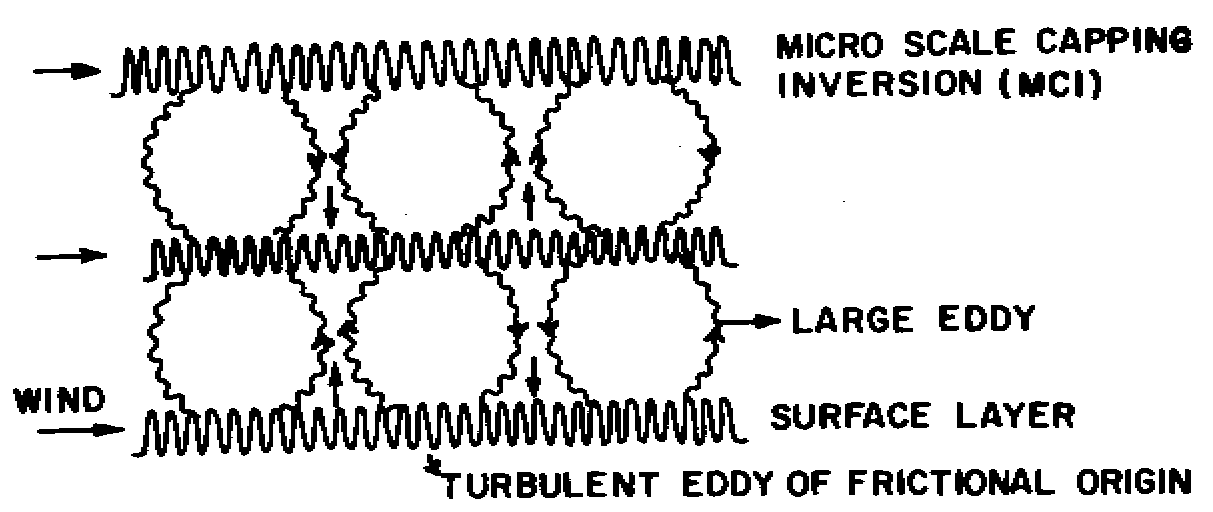
Figure 1 : Eddies in the PBL
The turbulent edies are carried upward
on the envelopes of the large eddies. Buoyant turbulent kinetic energy
generation by MFC occurs in the environment of the turbulent eddies
and gives rise to the formation of microscale capping inversion ( MCI
)
layer on the large eddy envelope. The MCI is seen as the rising
inversion of the day time PBL in echosonde and radiosonde records.
Townsend ( 1956 ) has
derived the following relationship between the large and turbulent eddy
root mean square ( r.m.s ) circulation speeds W and
w*
and their respective radii R and r as follows
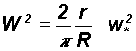
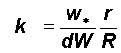
where w* is
the turbulent vertical velocity production by MFC and dW
is the corresponding increase in the large eddy circulation speed. The
value of k is greater than 0.5 for length scale
ratio z less than 10 . Though a continuous
spectrum of eddies propagate outward from the planetary surface , only
those eddies with length scale ratio greater than or equal to 10
can exist as discrete, identifiable, semipermanent entities in the PBL
since dilution by vertical mixing erases their( large eddy ) signature
for smaller scale ratios. In summary, the PBL contains a semipermanent
hierarchical system of eddies consisting of the convective, meso-, synoptic
and planetary scales which evolve basically from the dominant turbulence
scale at successive decadic scale range intervals and is manifested as
mesoscale cloud clusters and cloud rows in global synoptic weather systems.
Enhanced condensation inside clouds amplifies the myriads of turbulent
eddies and gives rise to cloud top gravity oscillations. Cloud water condensation
in the innumerable turbulent eddies is responsible for the observed cauliflower-like
surface granularity of the cumulus cloud. The physical mechanism of growth
of the atmospheric buoyancy ( gravity ) waves from turbulent buoyant energy
production is analogous to the conditional instability of the second kind
( CISK ) mechanism ( Holton, 1979 ) where hurricane systems are postulated
to derive their energy from convective scale cloud condensation. Also there
is an inherent two-way energy feedback mechanism in the hierarchical system
of eddies discussed in this paper and given in Equation 1 which is a statement
of the law of conservation of energy, selfsimilarity and self-consistency
in atmospheric processes. The kinetic energy E of unit volume
of the atmospheric eddy of frequency n
may be shown to be equal to Hn
where H is the instantaneous spin angular momentum of unit
volume of planetary scale eddy about the earth's axis. It is further shown
( Mary Selvam, 1986 ) that the eddy energy spectrum gives the probability
density distribution of the eddy field. Thus, the physical laws governing
eddy dynamics in the macroscopic planetay atmosphere is analogous to the
quantum mechanical laws of the sub-atomic space. Therefore the mesoscale
cloud clusters are a visible macroscale manifestation of the universal
quantum mechanical nature of the energy stucture of natural phenomena.
The full continuum of atmospheric eddies exist as a unified whole in time
and space and contribute to the manifested atmospheric phenomena in the
global planetary atmosphere and such a concept is similar to the bootstrap
theory of Chew ( 1968 ) and the theory of implicate order envisaged
by Bohm ( 1951 ).
The relationship between
the size ( R ) , time period ( T ), circulation
velocity ( W ) and energy ( E ) scales of the
convective ( c ) , meso- ( m ), synoptic ( s ) and planetary ( p ) scale
atmospheric eddy system to the basic turbulence scale ( t
) is derived from Equation 1 and is given below.
R : Rt = r : 10r : 10
2r
: 10 3r : 10 4r
T : tt = t : 40t : 40
2t
: 40 3t : 40 4t
W : Wt = w*
: 0.25w* : 0.25 2w* : 0.25 3w*
: 0.25 4w*
E : Et = e
: 62.5 e
: 62.5 2e
: 62.5 3e
: 62.5 4e
The globally observed
quasi-biennial oscillation ( QBO ) and the 20-year cycle in weather patterns
( Burroughs, 1986 ) may possibly result respectively from the fundamental
semi-diurnal atmospheric oscillation ( QBO ~ 12 hrs x 402 )
and the 5 - minutes oscillations of the sun's atmosphere ( 20 years ~ 5
minutes x 404 ) ( Equation 3 ). Such a process is analogous
to anti-Stokes laser emission triggered by laser pump.
2.1 Wind Profile in
the PBL
The large eddy
growth occurs in the vertical across unit cross-section of the large eddy
envelope in length steps dR equal to the turbulent eddy radius
r
since it is during this upward turbulent displacement of air parcels in
the MCI that a large eddy circulation velocity increase
dW
per second occurs because of turbulent buoyant energy production by MFC
. The circulation velocity W of a large eddy of radius
R
which begins growth from turbulence scale r from the planetary
surface is therefore obtained from Equation 2 as
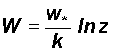
This is the well known
logarithmic wind profile relationship in the surface ABL and the
Von
Karman's constant k is now defined by the new theory
as representing the fractional volume dilution rate of the large eddy by
vertical mixing due to turbulent eddy fluctuations for a scale ratio of
10
. Further, the theory states that the logarithmic wind profile relationship
prevails throughout the PBL , the absolute value of W
being determined solely by MFC in the dominant turbulent eddy. Logarithmic
spiral airflow tracks ( vortices ) are therefore associated with vortex
roll circulations and is consistent with observations ( Hauf, 1985 ). The
atmospheric eddy continuum therefore consists of a hierarchical system
of vortices within vortices.
The parcels of air
rising up from the surface in the updraft regions of large eddy circulations
get diluted by vertical mixing and only a fraction f reaches
the normalised height z and is given by ( Mary Selvam et
al ., 1985 ).
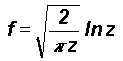
W = w* f z
Since the eddy energy
is derived from microscale fractional condensation on hygroscopic nuclei
in the troposphere the eddy enegy spectrum is dependent on the atmospheric
nuclei size spectrum. The observed atmospheric nuclei spectra follow the
Junge aerosol size spectrum ( Pruppacher and Klett, 1978 ) and it is shown
that the observed aerosol size spectrum is a natural consequence of the
atmospheric eddy continuum ( Ramachandra Murty et al ., 1985 ).
The aerosol size spectrum has a computed maximum spectral slope of -4.8
in the maximum size region.
2.2 Atmospheric Eddy
Energy Spectrum
The atmospheric eddy
energy spectra obtained by observations of turbulence spectra of wind in
the PBL show the existence of a continuous spectrum of eddies with
universal characteristics of scale invariant spectral slope ( Nastrom and
Gage, 1985 ).
The atmospheric eddy
energy spectral slopes Sd and Sm corresponding
respectively to dry and moist atmosphere are computed ( Ramachandra Murty
et
al ., 1985 ) and shown to agree with observations.
Selfsimilarity and
scale invariance are implied in atmospheric processes since the eddy energy
spectrum follows a power law of the form n-n
where n
is the frequency and n is the exponent. It is therefore theoretically
possible to predict weather trends and physical/chemical characteristics
of air motions at all scales provided n is known.
2.3 Synoptic Scale
Cloud Bands
The cloud bands identify
the circulation path of the synoptic eddy whose radial growth occurs in
legth steps dR equal to r and the corresponding
angular rotation dq
may be shown to be equal to f . Therefore in regions of weak
pressure gradients, r is large and dq
small and cloud rows occur. With increasing moisture supply, r
decreases and dq
increases resulting in tight coiling of cloud bands as in hurricane systems
( Mary Selvam, 1986 ).
The pressure and wind
anomaly patterns of synoptic eddy systems are universal with respect to
the normalised length scale and are shown at Figure 2.
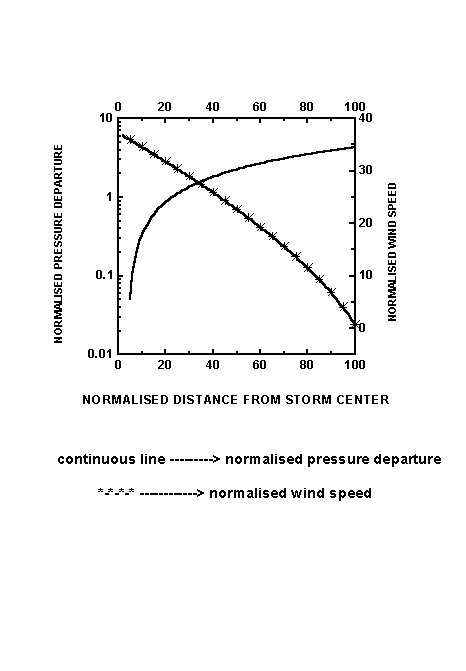
Figure 2 : Universal pressure and wind
anomaly patterns in synoptic eddy systems
Decrease in r results in
increase in absolute values of the pressure and wind gradients.
The above physical
mechanism envisaged for atmospheric eddy growth postulates the co-existence
of large and small eddies in a hierarchical system with ordered dynamical
local-global mutual energy exchange and therefore all energy systems are
inherently non-local. It is shown that such a concept leads to the universally
observed normal distribution characteristics in natural phenomena. Also,
Lovejoy and Schertzer( 1986 ) have established the fractal characteristics
of rain areas and rainfall in association with the observed scale invariant
characteristics of the atmospheric eddy energy spectrum. The regions of
eddy continuum energy enhancement are conventionally associated with ordered
chaos. The universal route to chaos, namely, period doubling, intermittency
and scale invariant eddy energy structure ( Harrison and Biswas, 1986 )
is commonly observed in all natural growth phenomena ( Fairbairn , 1986
) and therefore is an inherent characteristic of the quantum mechanical
nature for the eddy energy structure manifestation in natural phenomena.
Since eddy circulation inherently consists of opposite directions of motion
in association with different manifested phenomena, the apparent quantisation
of energy in nature occurs. Eddy motions or vibrations in the PBL
are responsible for the observed normal distribution characteristics of
thermodynamical parameters as explained in the following. The eddy energy
propagates by the inherent property of inertia of the medium and therefore
w*
, w*2 ,
w*3
and w*4 respectively represent
the inertia, force, angular momentum and spin angular momentum of the medium
caused by the eddy motion initiated by the turbulence scale acceleration
w*
per second. Therefore, the mean ( w ), variance ( s2
), skewness and kurtosis are respectively given by w*
, w*2 , w*3
and w*4 . The moment coefficient
of skewness is equal to zero. The moment coefficient of kurtosis
is equal to three and represents a factor of three increase in the spin
angular momentum for the inherent period doubling process of large eddy
growth in the MCI . Therefore the normal distribution characteristics
conventionally attributed to random chance are in reality the deterministic
laws of eddy growth by the period doubling process and represent the implicit
order in natural phenomena. Signal and noise exist in each other in nature
since signal is but the integrated mean of the conventional background
random noise over large space and time scales. Further, the following fundamental
relations in statistics and mathematics may be obtained from the physical
concept of the eddy growth mechanism as follows. The standard equation
relatining population and sample standard deviation follows from Equation
1, namely, W22 = W12
/n where n = z2 / z1 , and
z1,
z2
are the respective scale ratios with respect to the turbulence scale for
two large eddy circulations of root mean square circulation speeds W1
and
W2 respectively. The function
f
also gives the angular displacement of the particle trajectory on the large
eddy envelope for successive radial growth steps r and it
may be shown that f = p
= 22/7 = circumference/diameter
for a complete large eddy circulation of scale ratio 10 .
The hierarchical growth of large eddies from turbulence scale is basically
by a period doubling process in the MCI where incremental
growth occurs in length steps equal to r . The layer MCI
is thus a region of chaos. The unique particle trajectory design of concentric
circles in the field of chaos has been named the strange attractor and
results from the inherent hierarchy of the continuum vortex roll circulations.
For example, the strange attractor design is seen in the MCI where
maximum aerosol concentration occurs with a layered fine structure representing
the component turbulent eddies and has been observed commonly in the stratospheric
Junge aerosol layer, the arctic haze ( Radke et al ., 1984 ) and
more spectacularly in the planetary rings of Jupiter, Saturn and Uranus
( Michel, 1985 ) indicating dusty debris filled atmosphere and violent
equatorial convective dynamics for the major planets. Particles in the
planetary rings may be shown to follow the relation R3
/ T2 = constant from Equations 1 and 2
and is therefore consistent with Kepler's third law of planetary motion.
2.4 Cloud Microphysics
and Dynamics
Cloud growth occurs
in the updraft regions of vortex roll circulations in the low pressure
field of synoptic scale systems. From the theory of of atmospheric eddy
dynamics it is shown that (1) The vertical profile of the ratio of q
, the actual cloud water content to the adiabatic liquid water content
qa
follows the f distribution. (2) The vertical profile profiles
of the vertical velocity W and the total water content qt
are respectively given by W = w* fz and qt
= q* fz where t represents the total values
and * represents cloud base values. (3)
The cloud growth time
T = [ li {sqrt(z)}]2z
where li is the logarithm integral. (4) The cloud
drop size spectrum follows the Junge aerosol size spectrum and (5) The
computed raindrop size spectrum closely resembles the observed Marshal-Palmer
raindrop size distribution at the surface ( Mary Selvam et al .,
1985).
3.0 Planetary Atmospheric Electrification
3.1 Fair Weather Electric
Field
The atmospheric eddy
continuum circulations give rise to vertical mass exchange in the ABL
such that a net positive space charge current flows upward with a simultaneous
downward transport of negative space charges from ionospheric levels and
this dynamical two-way charge transport is shown to be of the right order
of magnitude and direction to sustain the fair weather atmospheric electric
field and also explain the horizontal component of the geomagnetic field
distribution ( Mary Selvam et al ., 1984b ). The above theory also
helps to explain the observed ( Gribbins, 1981 ) close similarity between
the geomagnetic field lines and atmospheric circulation patterns. Therefore,
changes in atmospheric circulation patterns preceding climate changes can
be detected in geomagnetic field pattern variations. The wandering of the
geomagnetic north pole is therefore closely related to global climate variations
and incidentally is also reflected in the subatomic dynamics of ferromagnetic
substances which naturally align themselves along geomagnetic N-S direction.
3.2 Cloud Electrification
It is shown that cloud
top gravity oscillation mix overlying environmental air into the cloud
such that there is a downward transport of negative space charges from
above cloud top regions and a simultaneous upward transport of positive
space charges from below cloud base levels to the cloud top regions. Positive
dipole cloud charging occurs by the vertical mixing. The electric field
at the surface due to the cloud dipole charge, the strength of the cloud
dipole, the cloud electrical conductivity, the corona discharge current
are expressed in terms of the basic non-dimensional parameters f
and z ( Mary Selvam et al ., 1984b ).
4.0 Cloud Dynamics and Urban Effects
The thermal energy input
from industrial/urban sites in combination with hygroscopic nuclei and
moisture lead to enhanced cloud growth process with taller clouds and heavier
rainfalls
particularly in the downwind region. A fraction f of the
surface nuclei form cloud/raindrops and therefore the same fraction
f
of atmospheric pollution content will also get washed down in the rain.
Even in clear weather conditions the pollution of atmospheric air in the
form of aerosols will be equal to a fraction f of the value
at the source location. The steady state flux of pollution transport can
therefore be taken to be given by the f distribution both
in the vertical and horizontal. Though f is small at large
values of the normalised distance z , yet long-term accumulations
of pollution will be appreciable resulting in irreversible environmental
degradation. Also, enhanced dynamics associated with thermal energy supply
from the urban industrial sites leads to a faster transport of pollutants
in all directions.
One possible solution
to prevent soil and rain water acidification is to release alkaline mineral
dust ( natural soil ) along with the chimney gaseous effluents since cloud
drops form on large aerosols and therefore rainwater acidificaion may be
prevented. Such instances of inadvertent neutralisation of acid rain in
the meditteranean/ European countries has been observed in association
with Saharan dust episode resulting from the African drought and the El-Nino
( Loye-Pilot et al ., 1986 ). Also, Indian region rainfall even
in close vicinities of industies is found to have alkaline rain in association
with the alkaline nature of the dust content in the air ( Khemani et
al ., 1985 ).
5.0 Atmospheric Vortices
and Stratospheric Dynamics
Thermal energy sources are regions of enhanced eddy dynamics and vertical mixing extending to the stratosphere and above. Enhanced downward flux of stratospheric ozone occurs above regions of industrial/urban activity. Beig and Chakravorthy ( 1986 ) have reported a sharp decrease in stratospheric ozone in association with a major fire in an off-shore oil well in India. Downward transport of stratospheric ozone occurs in regions of deep convection ( Gushchin and Sokolenko, 1985 ) . Stratospheric aerosol and radioactive debris from volcanic eruption and nuclear experiments/accidents are transported downwards to surface levels in regions of deep convection where intense vertical mixing occurs. Such regions of stratospheric contamination deposition on surface even in fair weather will occur in discrete areas of fractal nature analogous to rainfall areas ( Lovejoy and Schertzer, 1986 ) and thus may account for the radiation hot spot fall out pattern reported following the Chernobyl nuclear reactor accident ( Nature, 1986 ). Also, the recently reported ozone hole in the Antarctic stratosphere ( Tung et al ., 1986 ) may possibly be caused by the increased international exploration activities in Antarctica during the spring/summer season in recent years.
5.1 Atmospheric Vortices
and Ionospheric Dynamics
It is known that solar
flares perturb the ionosphere and cause intensification of weather systems.
Therefore ionospheric heating experiments and the numerous earth orbiting
satellites may possibly create fine scale magnetospheric/ionospheric perturbations
and lead to inadvertent modification of climate, for example, the African
drought, anomalous El-Nino and abnormal hurricane activity.
6.0 Conclusions
Energy structure of
natural phenomena in the PBL are inherently scale invariant with
a two-way energy feedback mechanism between the larger and smaller scales.
Persistent local microscale modifications of the atmospheric composition
and energy structure is bound to be manifested in course of time in a magnified
version in the macroscale as global changes in atmospheric composition,
climate and dynamics. Urban/industrial activities are therefore unavoidably
linked with global climate change and environmental pollution, the latter
of which can possibly be averted by suitable preventive measures.
References
Beig, E. and D.K. Chakravorty,1986 : Fire threat to ozone. New Scientist, July 31, 25 .
Bohm, D., 1951 : Quantum Theory . Prentice Hall, New York, 614pp.
Burroughs, W., 1986 : Randomness rules the weather. New Scientist, 10 July, 36-40.
Chew, G.F., 1968 : 'Bootstrap' : A scientific idea? Science, 161, 762-765 .
Eymard, L., 1985 : Convective organisation in a tropical boundary layer. An interpresentation of Doppler radar observations using Asai's model. J. Atmos. Sci., 42, 2844-2855.
Fairbairn, W., 1986 : Organised chaos : A signature of non-linearity. Phys. Bull., 37, 300-302.
Gribbins, J., 1981 : Geomagnetism and climate. New Scientist Feb. 5, 350.
Gushchin, G.P. and S. A. Sokolenko, 1985 : Fluctuations of total ozone upon passage of tropical cyclones. Soviet Meteor. and Hydrology, 10, 36-42.
Harrison, R.G. and D.J. Biswas, 1986 : Chaos in light. Nature, 321, 394-401.
Hauf, T., 1985 : Rotating clouds within cloud streets. Contributions to Atmos. Phys., 58, 380-398.
Holton, J.R., 1979 : An Introduction to Dynamic Meteorology . Academic Press, New York, p.39.
Khemani, L. T., G. A. Momin, M. S. Naik, P. S. Prakasa Rao, R. Kumar and Bh. V. Ramanamurty, 1985 : Impact of alkaline particulates on pH of rainwater in India. Water, Air and Soil Pollution 25 , 365-376.
Loye-Pilot, M. D., J. M. Martin and J. Morelli, 1986 : The influence of Saharan dust on the rain acidity and atmospheric input to the Mediterranean. Nature 321 , 427-431.
Lovejoy, S. and D. Schertzer, 1986 : Scale invariance, symmetries, fractals and stochastic simulations of atmospheric phenomena. Bull. Amer. Meteorol. Soc., 67, 21-32.
Mary Selvam, A., A.S. Ramachandra Murty and Bh.V. Ramana Murty, 1984a : Role of frictional turbulence in the evolution of cloud systems. Proc., IX Int'l Cloud Physics. Conf., 21-28, Aug., Tallinn, USSR, 387-390.
Mary Selvam, A., A.S. Ramachandra
Murty, G.K.Manohar, S.S.Kandalgaonkar and Bh.V. Ramana Murty, 1984b : A
New mechanism for the maintenance of fair weather electric field and cloud
electrification. Proc.of VII Int.Conf.on Atmospheric Electricity,
4-8 June, Albany, NewYork, 154-159.
http://xxx.lanl.gov/abs/physics/9910006
.
Mary Selvam, A., and A.S.R. Murty, 1985 : Numerical simulation of warm rain precesses. Proc. 4th WMO Scientific Conf. Wea. Mod., 12-14 Aug., Honolulu, Hawaii, USA, 503-506. http://xxx.lanl.gov/abs/physics/9911021 .
Mary Selvam, A. 1986 : A gravity wave feedback mechanism for the evolution of mesoscale cloud clusters ( MCC ). Proc. Int'l Conf. Monsoon and Mesoscale Meteorology, 4-7 Nov., Taipei, Taiwan, 84-89.
Mathews, H., W. Kellog and G. D. Robinson, 1971 : Man's Impact on the Climate . MIT Press, Massachusetts, USA, 594pp.
Michel, F. C., 1986 : The collisionless rings of Uranus. Geophys. Res. Letters 13 , 442-443.
Nastrom, G. D. and K. S. Gage, 1985 : A climatology of atmospheric wave number spectra of wind and temperature observed by commercial aircraft. J. Atmos. Sci . 42 , 950-960.
Nature, 1986 : News, Chernobyl accident, Nature, 322, 765.
Pruppacher, H.R. and J. D. Klett, 1978
:
Microphysics of Clouds and Precipitation .D.
Reidel Publishing Co., Boston, USA, 714pp.
Radke, L.F., J. H. Lyons, I. H. Bailey and P.V. Hobbs, 1984 : Airborne measurements of the vertical distribution of Arctic haze. Preprints, 11th Int'l Conf. Atmospheric Aerosols, Condensation and Ice Nuclei, Sept. 3-8 Sept., Budapest, Hungary, Vol.I, 138-142.
Ramachandra Murty, A. S., A. M. Selvam, S. S. Parasnis and Bh.V. Ramana Murty, 1985 : Warm cloud dynamical response to salt seeding. Proc. of the 4th WMO Scientific Conf. on Weather Modification, 12-14 August, Honolulu, Hawaii, 489-492.
Tennekes, H. 1973 : Similarity laws and scale relations in planetary boundary layers. In: Workshop on Micrometeorology , Amer. Meteorol. Soc., Boston, USA, 177-216.
Townsend, A. A. 1956 : The structure of turbulent shear flow . Cambridge University Press, Cambridge, pp.115-130.
Tung, K. K., M. K. W. Ko, J. M. Rodriguez
and N. D. Sze, 1986 : Are Antarctic ozone variations a manifestation of
dynamics or chemistry? Nature 322 , 811-813.