2.1.1 Fractals in Pure Mathematics
Irregular (wrinkled) patterns are often described by functions that are continuous but not differentiable. Till the late 1800s pure mathematics dealt mostly with functions which are differentiable everywhere such as the circle or ellipse. Pioneers in the study of functions which are continuous everywhere but without tangents are Karl Weierstrauss (1815-1897) who presented the Weierstrauss function in 1872, George Cantor (1845-1918) who provided the Cantor set in 1883 and Helge Van Koch (1906) who first constructed the snowflake curve(Deering and West,1992 References)). A representative example,the Koch's curve is shown in Figure 2.
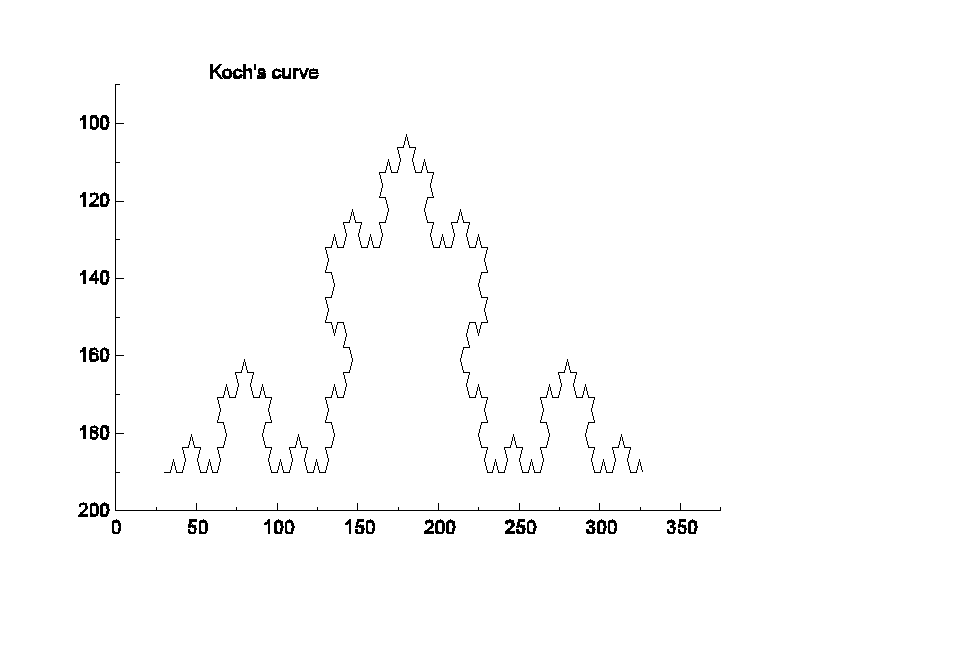
Jagged boundaries represented by these functions are more common in nature than the special case of curves with tangents, such as the circle. However, real world geometrical structures were not associated with these functions till a long time after their discovery. Continuous functions which are not differentiable anywhere represent an infinite number of zigzags between any two points. The length between any two points on the curve is infinity, yet the area bounded by the curve is finite. These "monster curves" which were outside the domain of pure mathematics were ignored as a field for study by many prominent mathematicians till the late 1800s.
The non-Euclidean geometry of the "monster curve" was quantified in terms of the similarity dimension by Hausdorff in 1919 References. His idea was based on scaling, which means measuring the same object with different units of measurement. Any detail smaller than the unit of measurement is discarded. The jagged "monster curves" have fractional (non-integer) dimensions. The word 'fractal' was coined by Mandelbrot(1977) as a generic name for such objects as Koch's snowflake which possess fractional Hausdorff dimension. Besicovitch(1929) References was a second major figure who had developed the background for the concept of fractional dimension. Some of the earlier studies on applications of scaling concepts are given in the following. The question of scaling and the paradigm of fractals,i.e., when can a part have the same propertiesas the whole was addressed in the 1920s and 1930s by Levy(1937) References who was concerned with the question of when a sum of identically distributed random variables has the same probability distribution as any one of the terms in the sum(Shlesinger et.al., 1987 References). The length of a fractal object,e.g. the coastline increases with decrease in the length of yardstick used for the measurement. Richardson(1960 References) came close to the concept of fractals when he noted that the estimated length of an irregular coastline or boundary B(l) ,where l is the measuring unit is given by B(l)=Bol1-d where Bo is a constant with dimension of length and d is the fractal dimension greater than 1 but less than 2 for the jagged coastline(West, 1990a,b References).One of the oldest scaling laws in geophysics is the Omori law(Omori, 1895 References).It describes the temporal distribution of the number of aftershocks which occur after a larger earthquake(i.e.,mainshock) by a scaling relationship.The other basic empirical seismological law,the Gutenberg-Richter law(Gutenberg and Richter, 1944 References) is also a scaling relationship,and relates intensity to its probability of occurrence(Hooge et. al., 1994 References).
The fractal dimension D in general for length scale R may be given as :
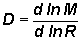 ----------- (1)
----------- (1)where M is the mass contained within a distance R from a point in the extended object. A constant value for D implies uniform stretching on logarithmic scale, resulting in large scale structures which preserve their original geometrical shape. Objects in nature are in general multifractals, i.e. the fractal dimension D varies with the length scale R. The multifractal nature of fluid turbulence and scaling concepts has been discussed by Sreenivasan(1991 References) The dimension of a naturally occurring fractal is a quantitative measure of a qualitative property of a structure that is selfsimilar over some regions of space or intervals of time.